Credit:
- Georgios Tsampras
- Richard Sause
- Dichuan Zhang
- Robert B. Fleischman
- Jose I. Restrepo
- David Mar
- Joseph Maffei
Published: Earthquake Engineering & Structural Dynamics, 2016
This paper presents the development of a deformable connection that is used to connect each floor system of the flexible gravity load resisting system (GLRS) with the stiff lateral force resisting system (LFRS) of an earthquake-resistant building. It is shown that the deformable connection acts as a seismic response modification device, which limits the lateral forces transferred from each floor to the LFRS and allows relative motion between the GLRS and LFRS. In addition, the floor accelerations and the LFRS story shears related to the higher-mode responses are reduced. The dispersion of peak responses is also significantly reduced. Numerical simulations of the earthquake response of a 12-story reinforced concrete shear wall example building with deformable connections are used to define an approximate feasible design space for the deformable connection. The responses of the example building model with deformable connections and the example building model with rigid-elastic connections are compared. Two configurations of the deformable connection are studied. In one configuration, a buckling restrained brace is used as the limited-strength load-carrying hysteretic component of the deformable connection, and in the other configuration, a friction device is used. Low-damping laminated rubber bearings are used in both configurations to ensure the out-ofplane stability of the LFRS and to provide post-elastic stiffness to the deformable connection. Important experimental results from full-scale tests of the deformable connections are presented and used to calibrate numerical models of the connections. Copyright © 2016 John Wiley & Sons, Ltd.
Related Research


FEMA P-58: Options For Earthquake Resistant Design

Conceptual Seismic Design Guidance for New Reinforced Concrete Framed Infill Buildings

Earthquake Safety Prioritization of School Buildings Using Performance-Based Risk Assessment in Kyrgyz Republic
Casa Adelante: Rocking Mat Foundation System

Development of Deformable Connection for Earthquake-Resistant Buildings to Reduce Floor Accelerations and Force Responses

FEMA P-807: Guidelines for Seismic Retrofit of Weak-Story Wood-Framed Buildings
Design Examples Using Mode Shaping Spines for Frame and Wall Buildings
Recommended Mesh Anchorage Details for Straw Bale Walls
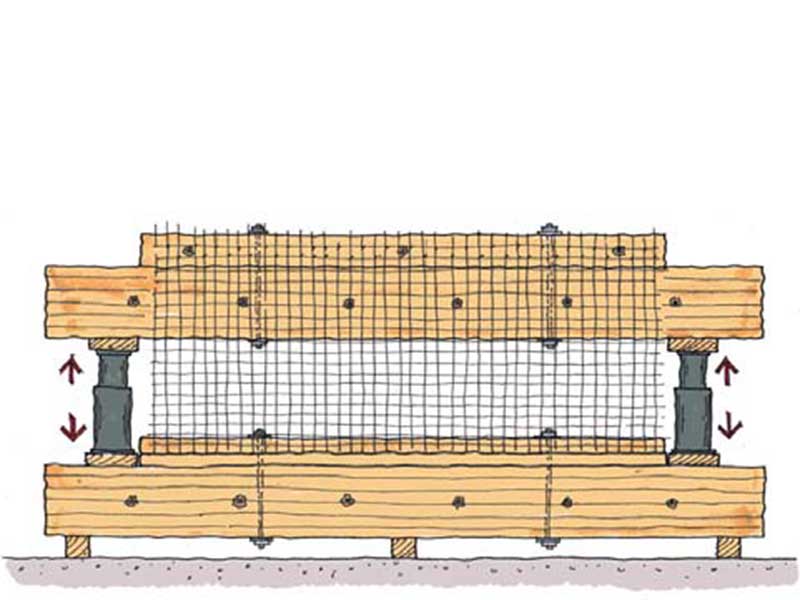
Reversed Cyclic In-plane Tests of Load-bearing Plastered Straw Bale Walls